What is a Tree ?

Contents

• What is a Tree ?
• What is an Edge?
• Type of Node
  • Root and Edge
  • Internal node and Leaf node
  • Parent, child and Sibling node
  • Descendant and Ancestor node
• Term of Node
  • Example of Level
  • Example of Height
  • Example of Degree
• Subtree

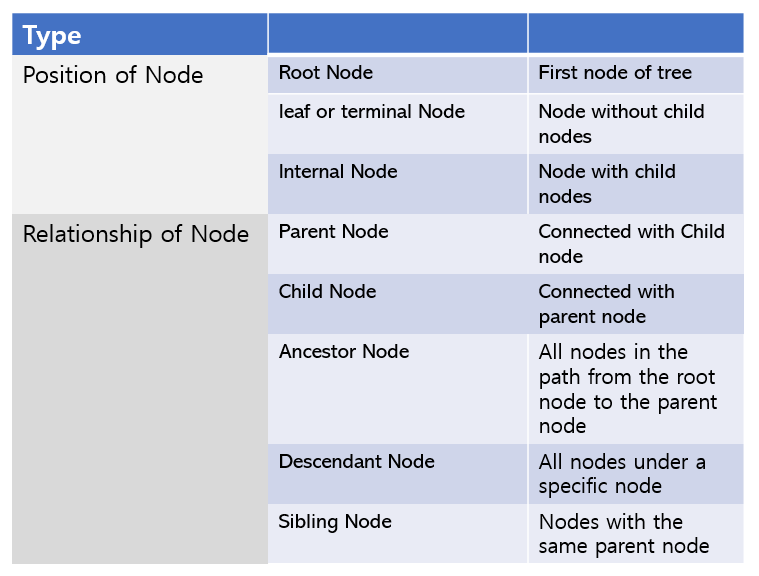
Data Structure Tree

What is a Tree ?

• A tree is a collection of node made up of hierarchical structures.
  • Parent-Child relationship
  • The next node can be multiple, but the previous node must be one.

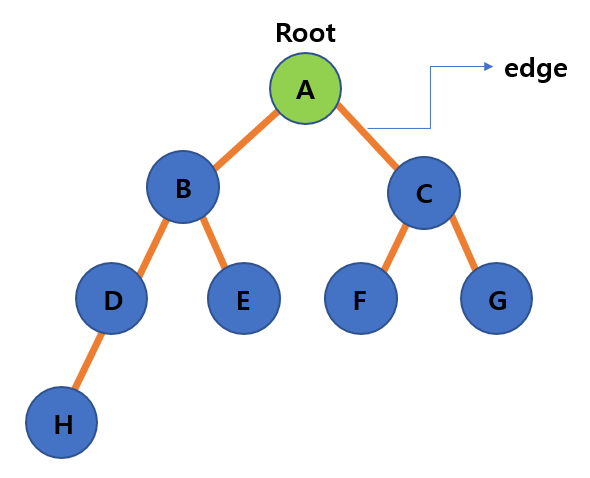
What is an Edge?

• A line that connects between nodes. The edge is used to indicate a parent-child relationship.

Type of Node

Node that are distinguished by the position of nodes in the tree.

• Root node - First node in the tree
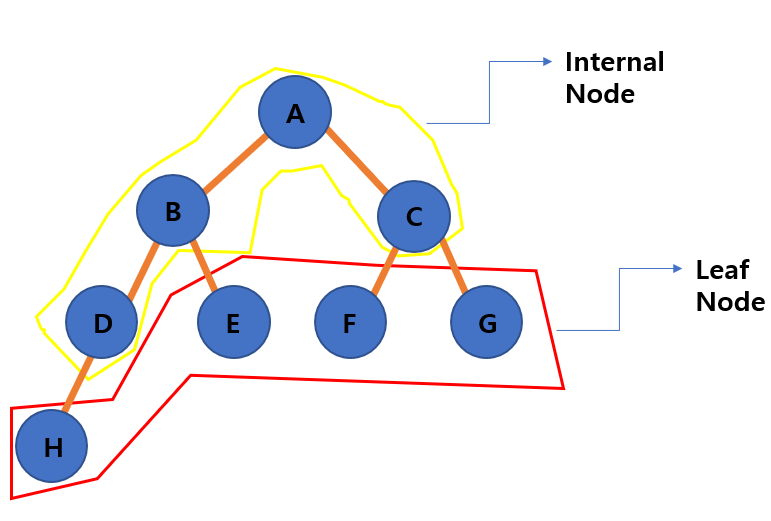
• Leaf node - node without a child node.
• Internal node - node with child node

Node that are distinguished by the relationship between nodes in the tree.

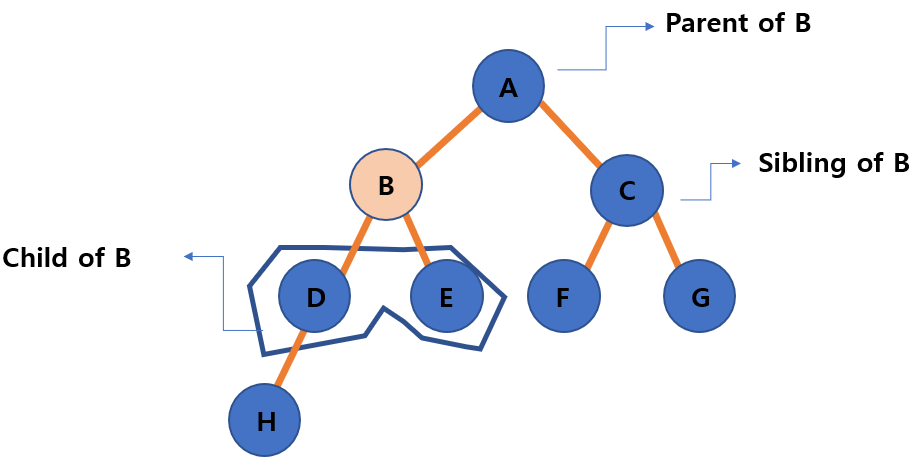
• Parent node - node connected directly above the child node
• Child node - node connected to the bottom of a node
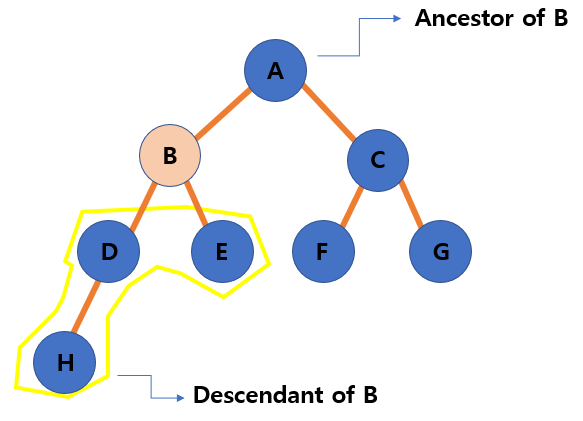
• Ancestor node - all nodes in the path from the parent node to the root node
• Descendant node - all child nodes associated with one node
• Sibling node - node with the same parent node

Root and Edge

Internal node and Leaf node

Parent, child and Sibling node

Descendant and Ancestor node

Term of Node

Terms of node

• Level - Distance from root node
• Height - The number of edges on the longest downward path between the root and a leaf
• Degree - Number of child nodes a node has

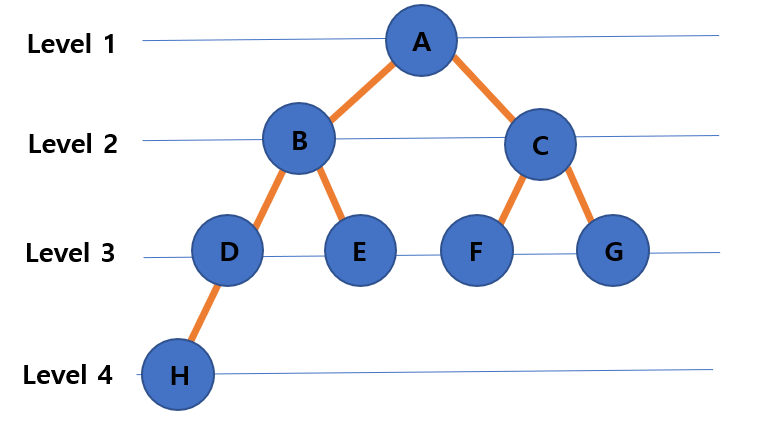
Example of Level

1. Because A is the root node, Level 1
2. Because B and C are 1 distance from the root node, Level 2
3. Because D,E,F, and G are 2 distance from the root node, Level 3
4. Because H is 3 distance from the root node, Level 4

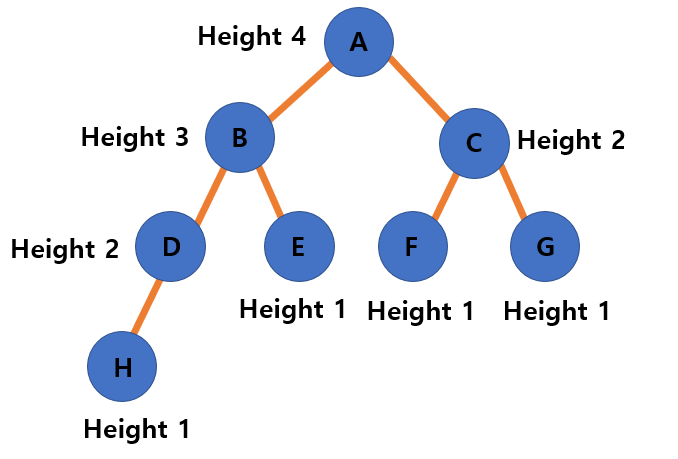
Example of Height

1. Because H has no children, Height 1
2. Because E,F, and G also have no children, Height 1
3. Because D and C have 1 edge to the furthest node, Height 2
4. Because B has 2 edges to the furthest node H, Height 3
5. Because A has 3 edges to the furthest node H, Height 4

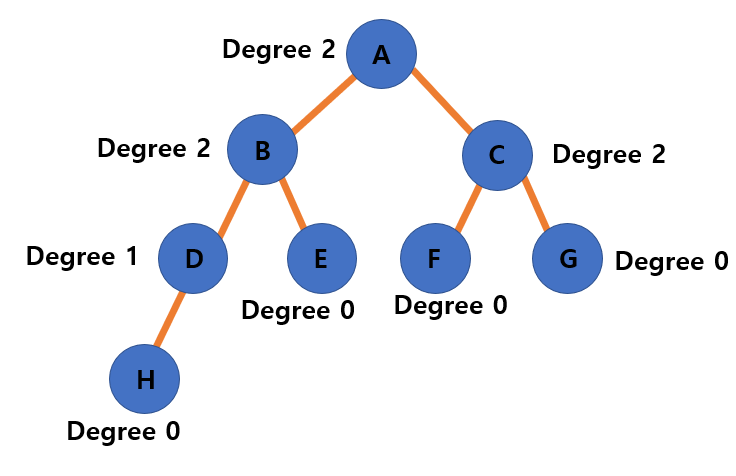
Example of Degree

1. H,E,F, and G have no children, Degree is 0
2. D has one child H, Degree is 1.
3. B and C have 2 children, Degree is 2.
4. A has 2 children, Degree is 2.

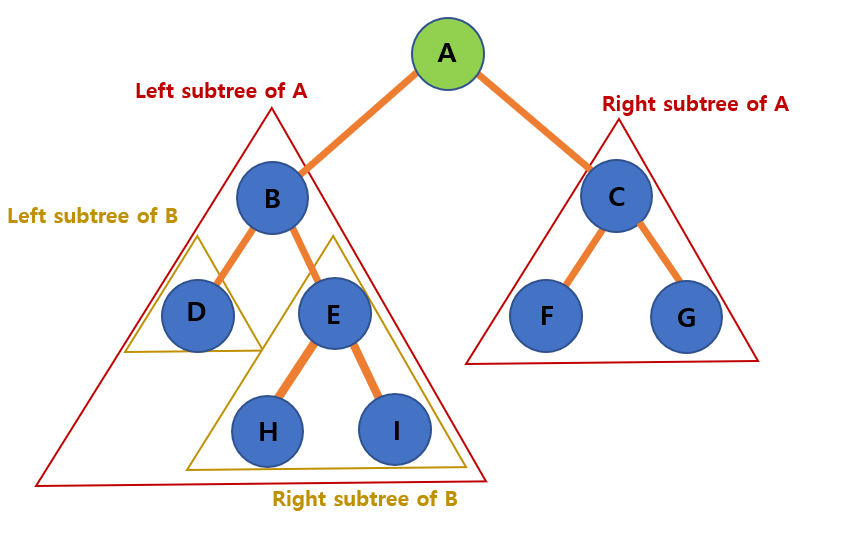
Subtree

• All nodes below a particular node form a left or right subtree.

  • The subtree on the left becomes ‘left subtree’
  • The subtree on the right becomes the “right subtree”